Zero-dimensional energy balance model of the climate system
Physics-based models of the climate system
(There are many types of models of the climate system, whose complexity is various.)
Zero-dimensional energy balance model
- The simplest physics-based model of the climate system.
- Governing equation: (only) conservation of energy.
- Spatial dimension: spatial variation in east-west, north-south, up-down dimensions are all ignored.
(Any quantity is assumed to be uniform in east-west and north-south directions,
and to have a constant profile except a single-value offset in the vertical direction.)
- Energy stock is assumed to be (constant) heat capacity multiplied by temperature.
- Energy input is absorption of solar radiation (mainly visible and near infra-red).
- Energy output is emission of terrestrial radiation (thermal infra-red).
- For convenience, quantities are expressed per unit area of the earth's surface.
- C dT/dt = (1/4) (1 - αp) S - Fout
- C: (effective) heat capacity of the climate system per unit area [J/(m2 K)]
- T: representative temperature of the climate system [K]
- 1/4: ratio between the cross-sectional area and the surface area of a sphere
- αp: reflectance of solar radiation by the earth as a whole,
or "planetary albedo". [non-dimensional]
- S: solar irradiance (energy flow per unit time per unit area)
at a plane perpendicular to the sun-earth line.
So-called "solar constant". [W/m2]
- Fout: radiance (energy flow per unit time per unit area) of
terrestrial radiation emitted to space [W/m2]
- Steady state
- T does not change in time. (S and αp are assumed to be constant as well.)
- 0 = (1/4) (1 - αp) S - Fout
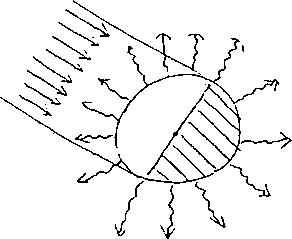
- Steady state, Assumption 1: Fout is blackbody radiation at temperature T.
- 0 = (1/4) (1 - αp) S - σB T4
- Assume that S = 1.36 × 103 W/m2 [Corrected after lecture], and αp = 0.30 (according to average values of modern observations).
- Then, T = 254 K = approx. -19 ℃
- This T is the representative temperature of the terrestrial radiation.
(Note: Terrestrial radiation in the real world is a mixture of radiation emitted
by the land and sea surface, by cloud top, and by atmospheric gases.
It is not uniform in space.)
This T is called "effective radiative temperature of the earth" Te.
- Steady state, Assumption 2: Fout is {blackbody radiation at temperature T} multiplied by εp.
- 0 = (1/4) (1 - αp) S - εp σB T4
- εp may be (temporarily) called "apparent planetary emissivity".
- εp T4 = Te4
- εp(1/4) T = Te
- T = Te / εp(1/4) = εp(-1/4) Te
- If εp < 1, T > Te.
- Smaller εp ... "stronger greenhouse effect"
- If T = 287 K (14 ℃, actual global average surface air temperture), how much is εp ?
A bath-tub model
- A stock-and-flow system as well as a feedback system
- Useful for explaining gradual change between quasi-steady states
- Governing equation: conservation of mass of water
- Stock: mass of water, proportional to water level
- Inflow: mass flow of water, independent of water level
- Outflow: mass flow of water, increasing function of water level
- For a given constant inflow and a given outflow function, a steady state exists.
From any non-steady state, the system will approach the steady state.
- What will happen if the inflow changes suddenly, and then kept at the new constant value?
- What will happen if the outflow function changes suddenly, and then kept at the new constant value?
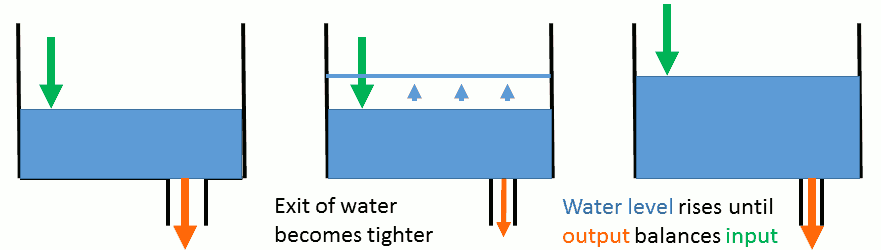
Analogy of the climate system with the bath-tub model (preliminary)
- Stock: energy stock, proportional to temperature
- Inflow: absorbed part of incoming solar radiation
- Outflow: outgoing terrestrial radiation
- "Water exit tightened" ... εp decreased ... "greenhouse effect enhanced".
Causes of global climate change that can be represented by 0-dimensional energy balance models
- Note: There may be causes of climate change that cannot be represented by 0-dim. models.
- Change of inflow of energy
- Change of energy flow emitted from the sun (Change of S)
- Change of matter in the space between the sun and the earth that interacts with radiation (unknown, but perhaps not important)
- Change of geometric configuration between the sun and the earth (important in 10000-year time scale)
- Change of absorption and reflection of solar radiation by the climate system (Change of αp)
- Change of outflow of energy
- Change of "apparent planetary emissivity" εp, in other words, change of "greenhouse effect"
What causes change in reflectivity of solar radiation?
How they depend on temperarture?
- Surface of land and ocean
- Snow and ice (feedback [to temperature])
- Vegetation, land cover (external forcing [as a 1st approx.])
- Suspended liquid and solid particles in the atmosphere
- Clouds [H2O] (feedback)
- Aerosols (external forcing)
What causes change in "apparent planetary emissivity" of terrestrial radiation? ...
- Gaseous components of the atmosphere ("greenhouse gases")
- Water vapor [H2O] (feedback)
- Carbon dioxide [CO2] etc. (external forcing)
- Suspended liquid and solid particles in the atmosphere (same as above)
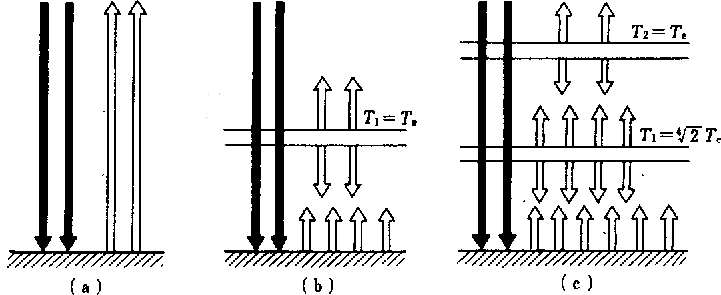
A "plate glass" model of the "greenhouse effect" of the atmosphere
- (Note that this is not the dominant effect of the actual greenhouse made of plate glass.)
- Zero-dimensional energy balance model, steady state.
- Sum of 2 black arrows corresponds to (1/4) (1 - αp) S .
- The ground is assumed to be blackbody.
- (a): the same as "assumption 1" above. Ground temperature T = Te.
- The atmosphere is assumed to be concentrated in a plate whose vertical dimension is negligibly small.
Also, it is assumed to be transparent for solar radiation and opaque (same as blackbody) for terrestrial radiation.
- (b): one plate
- Temperature of the atmospheric plate = Te
- Ground temperature / Te = 2(1/4) = sqrt(sqrt(2)) = approx. 1.2
- (c): 2 plates ... Ground temperature = 3(1/4) Te.
- More plates, higher ground temperature.
- The surface temperature of Venus (ca. 740 K) can be explained this way.
- This model can produce indefinitely high temperature, but that is not real,
because the separation of wavelength between solar and terrestrial radiation
will not hold at very high ground temperature.
2019-May-16
MASUDA Kooiti



